Physics of Diving
What happens to gases under water?
Boyle and the general gas equation
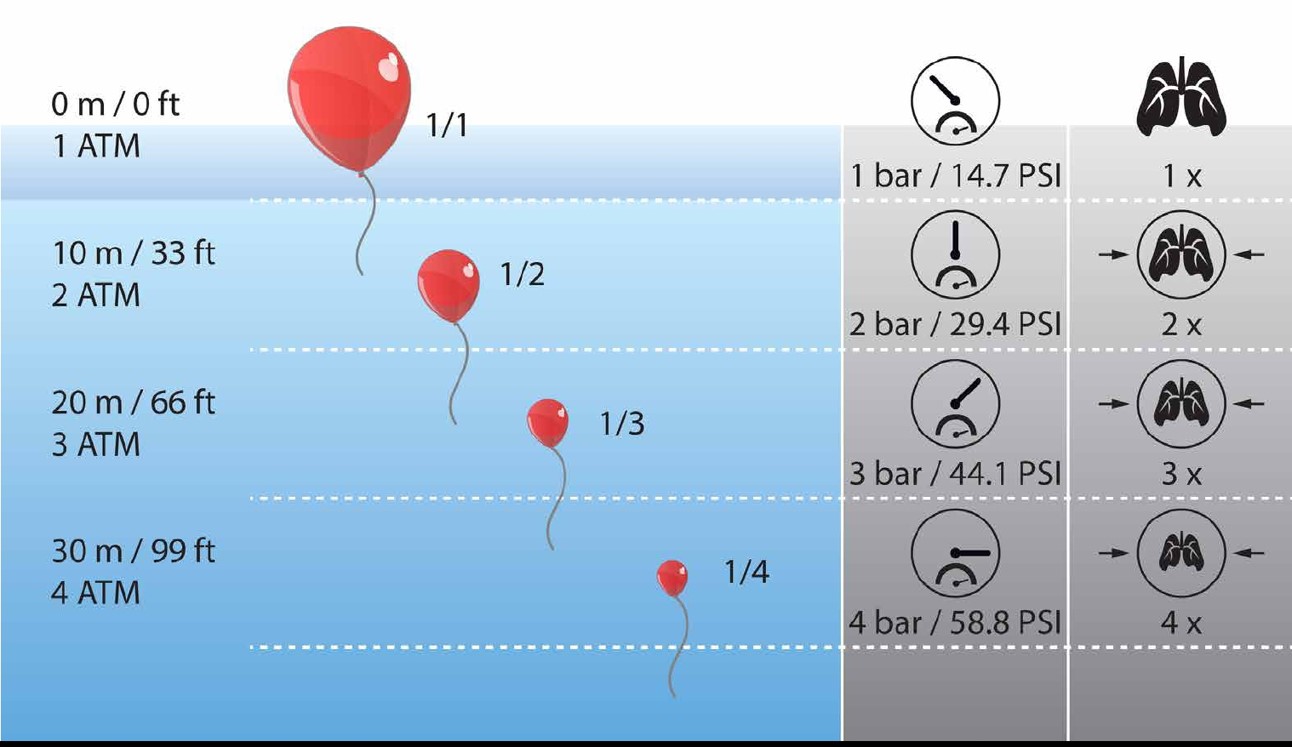
Right at the beginning of the OWD course, sometimes already at the taster dive, there is talk of Boyle: Pressure, Volume, Density. In short, gas volume and pressure have an inversely proportional relationship. When the pressure increases, the volume decreases. And if the pressure falls, the volume increases again. The density of the gas is proportional to the pressure, the same gas pressed to a smaller volume is of course correspondingly denser.
In principle, this is quite sufficient for almost everything you need to know about diving. However, it would only be complete if a few more components were also looked at.
As is often the case in physics, the quantities we are interested in can be put into a simple context. We call this relationship the “ideal gas equation”. Like all models, it is not perfect, and works with some simplifications. It considers our breathing gases to be “ideal” gases, where the particles of the gas neither attract nor repel each other, and where these particles themselves do not occupy space. Of course, this is obviously not quite correct for a real gas, but nevertheless this equation gives results accurate enough for a long and happy diving life!
If we look at this equation below, we recognize on the left side the quantities already known from Boyle’s law. On the right side, however, there is now the temperature T, a constant R, which is known from experiments and which represents the proportionality of the individual quantities, and the so-called quantity of substance n. Physicists and chemists measure this amount of substance in the unit “mol”, and one mol corresponds to the enormous number of 602 quadrillions of individual gas particles.
The wonderfully simple thing about this equation, however, is that in many cases — and so also in the case of the balloon whose volume at different ambient pressures we look at in the figure on this page — neither temperature nor particle number change appreciably. And if this is so, then of course we see immediately that also according to the ideal gas equation the product of pressure p and volume V is constant overall. This equation thus contains Boyle’s law, so to speak, but at the same time makes it possible to look at cases in which the temperature and amount of substance also change with time. And this is very valuable for us when diving, because after all, neither water is always the same temperature, nor do we want to leave the beautiful breathing gas in our tanks forever!
How important is the temperature?
For most questions involving gases under water, we can leave temperature out of the equation. But if you’ve ever wondered why the Fini suddenly reads less after you’ve gone into the water, you’ve already learned about another physical concept: Gay-Lussac’s law.
( pxV )/T=constant
When gases heat up, they expand. When they are in a closed container – i.e., a diving bottle, for example – they cannot expand; instead, the pressure increases.
What happens when you jump into cold water?
Suppose the air is 20 degrees and the water is only 10. I have 200 bar in the bottle.
Solution
The volume of the bottle does not change, you can simply omit it.
You need the temperature in Kelvin: You have to calculate from absolute zero, 0 degrees Celsius are about 273 Kelvin. 10 degrees Celsius would be 283, 20 degrees then 293 Kelvin.
200 bar/293K= x bar/283K
200bar/293Kx283K=193bar
When does the bottle become dangerously hot?
Let’s say we put a steel cylinder in the sun that is approved for 232 bar operating pressure (and tested at over 300 bar).
If it has 200 bar at 20 degrees room temperature, at what temperature does it reach the 232 bar?
Solution
We remember: p1/T1=p2/T2
Temperature: in Kelvin, 20 degrees Celsius – 293 Kelvin
In short, this is converted
p2xT1/p1=T2
232barx293K/200bar=339.8K – 66.8 degrees Celsius

What happens when something goes into the water? How does the salinity of the water affect my lead levels? How do gases behave under water?
Here we would like to apply some basic physics to things we might encounter underwater. Most of this is explained in the SSI Science of Diving Specialty, which you can supplement with our application examples.
How much does an anchor weigh?
Eureka! What a bathtub reveals about diving
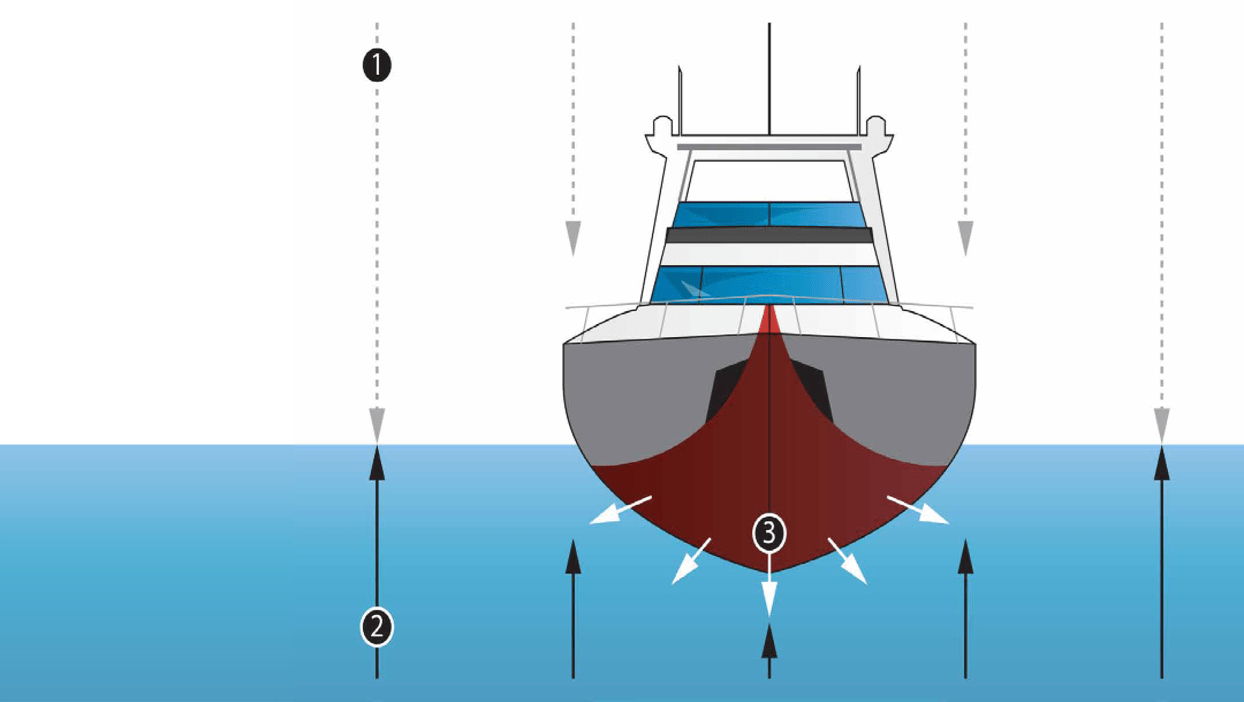
Archimedes – that ancient Greek with the geometry, the one with the Pi – is said to have been given a difficult task. He should find out whether a crown was made of real gold or a fake made of a cheaper material.
In search of a solution, the old man first took a bath. In the process, he thought about why the water overflows when he sits in it – and discovered the “Archimedes Principle” that is still known today.
Excited with joy, he is said to have run naked through the neighborhood after this discovery, shouting “Eureka”.
So what is the Archimedean principle?
The static buoyancy of a body in a medium is equal to the weight of the medium displaced by the body.
This means that everything is easier under water. The water we displace keeps us on top. We must exert as much force, that is, bring as much weight, as the water we displace weighs. That’s why we have so much lead, and that’s why we’re weightless under water: humans are 80% water.
Now what does that have to do with my anchor?
Such an anchor on the seabed is quite stable there: Made of metal, heavy and compact, it holds quite a bit. Anyone who has ever anchored a boat knows how small they are compared to the boat – and that anchors from large ships are significantly larger than what you use to moor a Zodiac.
Anchors in recreational boating weigh from 2 to 60 kg – 10kg is recommended for a 7.5m inflatable boat, and an 18m sailboat should carry at least 24kg. More precisely it is written here.
Nevertheless, you can’t just weigh an anchor on land and then know what its downforce is. From the weight of the anchor you need to subtract the weight of the water it displaces – so you need to know its volume.
Anchors are made of heavy metals, e.g. steel. Steel has a specific gravity of 7.85g, or just under 8g per cm³ – almost 8kg per liter. So a 24 kg anchor also displaces about 3l of water, so it only has about 21l of downforce.
To bring this anchor to the surface, we need to balance the downforce (not the weight!). In a lifting bag we need to fill 21l to balance the downforce and lift the anchor.
Whether we do it in salt or fresh water is only a theoretical issue. That comes in the next section…

Taring
When diving: buoyancy control
When diving, you initially adjust your mass with the lead, then the volume with gases.
Goal: Be exactly as heavy as the water you displace – then you are neutrally tared.
What sounds so simple, however, has some pitfalls: Choosing the right amount of lead at any given moment is not so easy. Even if you dive with your own equipment and know how much lead you need in a particular body of water, you will need to adjust the amount in other places around the world. Why? Firstly, because the water has a different salt content, and secondly, because bottles have different weights.
Influence of fresh and salt water
The seas and oceans around the globe contain relevant amounts of salt, unlike inland lakes. Exactly how much, however, can vary greatly.
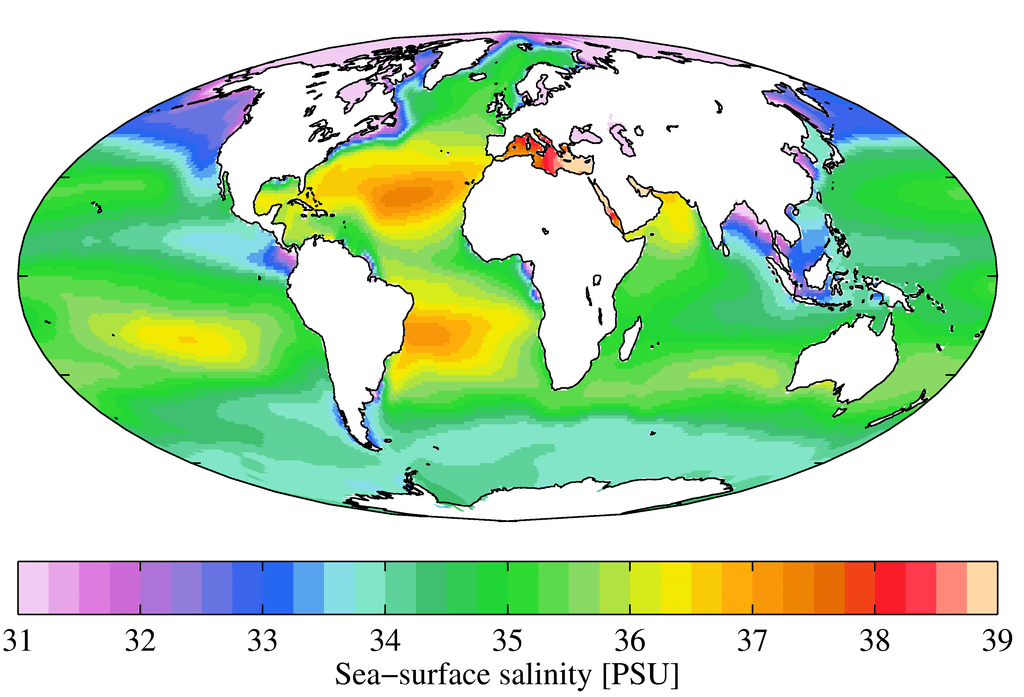
On the graph you can read the approximate salinity of a region. What is striking here is how low it is in Arctic waters – the reason for this is the meltwater from the ice cap bringing in fresh water. The more ice melts, the more clearly the salinity of the water changes – with consequences that are not yet foreseeable.
Around the equator, salinity is significantly higher, reaching the highest levels in the world in inland seas such as the Mediterranean or the Red Sea. The reason for this is the higher heat: the water evaporates and salt remains.
At the points where large amounts of freshwater from rivers reach the oceans, this pattern is interrupted and a much lower salinity is measurable.
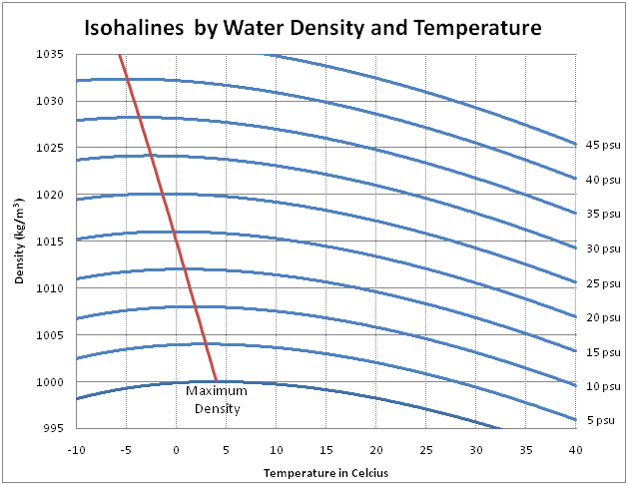
With the salt content alone, however, it is not yet clear how much heavier each liter of water is: After all, the dissolved salt also takes up a little space. To know this a little more precisely, let’s take a look at what the density of salt water with different salinities is at different temperatures. Fresh water has the highest density at 4 degrees Celsius, salt water at slightly lower temperatures depending on the salt content.
In the graph, you can read off the salt content on the right, then follow the line until you have the water temperature at the bottom in which you will be diving – and then read off the density on the left.
For example: when diving in the Canary Islands, the water contains around 35g of salt per liter. The water has about 20 degrees, the line here points to a density of 1025 kg/m³, i.e. 1.025 kg/l – this means that for each liter of volume you need 25g more lead than in fresh water.
The crux with the bottles
One might think that once the salt issue has been resolved, it would be easy to calculate what lead would be needed at a new spot. You can – if you do not also use a different bottle than usual.
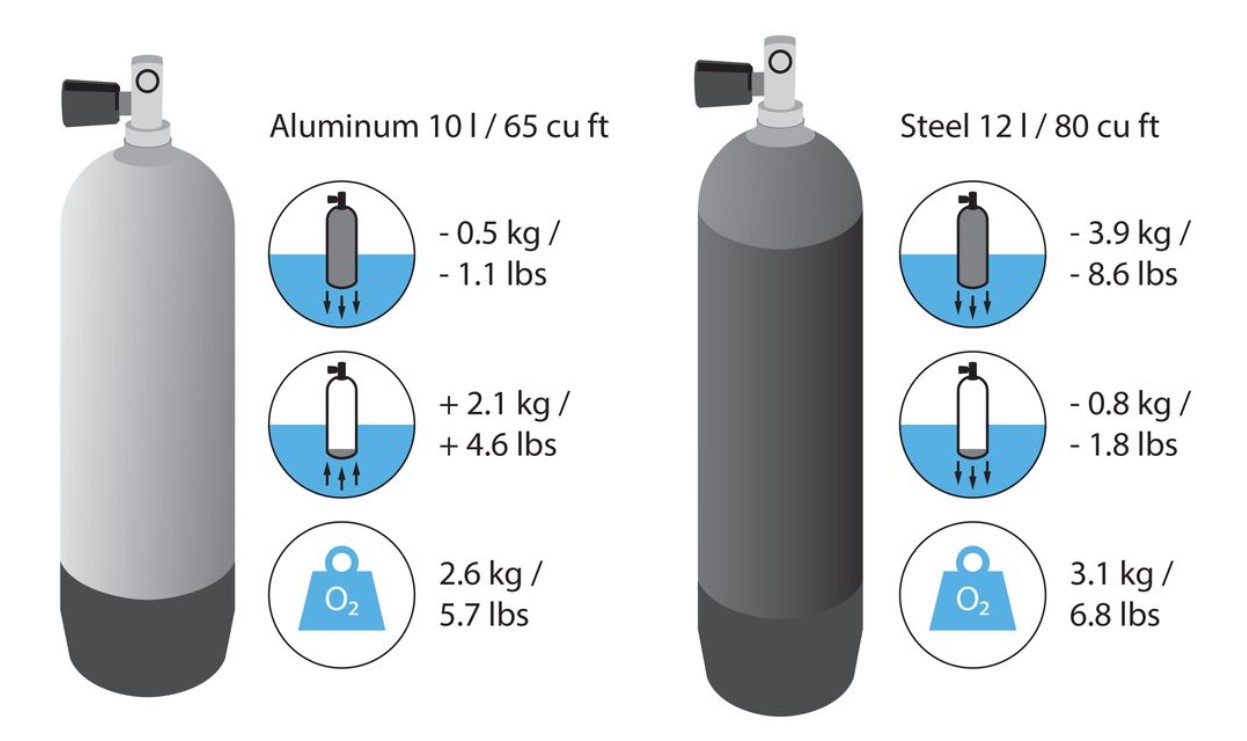
While steel cylinders are more popular in regions where diving is done in relatively cold water, aluminum cylinders are primarily found in tropical diving areas. Why?
Steel cylinders have downforce under water, even empty. Aluminum bottles, on the other hand, are more or less neutral; when empty, they have buoyancy. When diving in thick suits and needing a lot of lead, it makes sense to get some of the downforce from the tanks. In thin suits or lycras, on the other hand, a steel cylinder can be too much weight, aluminum is better for diving (and requires less maintenance…).
And then with bottles, it’s not just about what happens to them underwater; their weight on land is also interesting. Aluminum cylinders have much thicker walls than steel cylinders and are slightly heavier on average on land, although they have less downforce in the water. So you have to carry the higher weight of the bottle plus the additional lead – no one does that voluntarily.